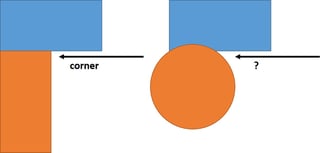
The intersection of two rectangles is called a corner, but is there a word for the intersection of a rectangle and a circle? Is it still a corner?
This picture I made probably explains it better:
(Obviously the reason I ask this is because a circle cannot/does not have any corners.)
corner:
the point at which two lines or edges meet[Longman]
The circle does not have any corner, but the new composite shape does. The first line comes from the circle, the second line comes from the rectangle, and they meet. By the above definition, the meeting point is a corner.
Another example is the corner of a room: even if the walls are curved or angled, you'd still call their intersection a corner.
I like corner, but here is an alternative.
The shape can be described as a half-cusp. A cusp can be generally used for any sharp point, but more specifically refers to the point where two curves meet. The shape reminded me of a cardioid chopped off at the cusp.
A cardioid (from the Greek καρδία "heart") is a plane curve traced by a point on the perimeter of a circle that is rolling around a fixed circle of the same radius. It is therefore a type of limaçon and can also be defined as an epicycloid having a single cusp.
