26

Is there any difference between the verbs reoccur and recur?

Several sources suggest that they are synonymous, but some fine-tuners suggest that there is a nuanced difference, such as Grammarist, which explains

Something that recurs happens repeatedly, perhaps at regular intervals. Something that reoccurs happens again, but not necessarily repeatedly or at regular intervals.

Does this rule exist, based on usage?

Also, according to this distinction, is the first recurrence of something strictly a reoccurence and not a recurrence? That seems a little incredible.

Improve this question
2
  • I would need more time to make a proper answer based on usage but my impression from a few COCA searches is that this distinction does not exist in practice.
    – z7sg Ѫ
    Commented Jul 12, 2011 at 14:01
  • The answer is yes. Think of a recurrent reminder in your desktop calendar program. Reoccur just means "occur again", not again and again.
    – Drew
    Commented Jul 25, 2015 at 4:05

5 Answers 5

Reset to default
19

Your quote from Grammarist matches what I would have answered. Expanding on it a little, I would use recur for an event that is expected to happen repeatedly or regularly and reoccur for an event that might happen again, but is not expected to.

The U.S. presidential elections recur every four years, but no one expects voting issues like the Bush vs. Gore election to reoccur anytime soon.

Improve this answer
8
  • If you expected it to happen exactly once more in the future, which would you use? For argument's sake, let's say you expected it to happen at a regular interval exactly once.
    – WAF
    Commented Jul 12, 2011 at 13:58
  • 1
    I would say 'elections occur every four years'.
    – z7sg Ѫ
    Commented Jul 12, 2011 at 14:05
  • @z7sg I started to write "The U.S. has recurring presidental elections every four years...", but I decided to rephrase it to use recur instead of recurring for a better fit with the question.
    – jimreed
    Commented Jul 12, 2011 at 14:09
  • @WAF I would use reoccur for an event that I expect to happen again exactly once.
    – jimreed
    Commented Jul 12, 2011 at 14:10
  • 1
    @jimreed I think it's better to avoid contrived example sentences if possible. Can you find an example of occur and reoccur from the same source? I doubt that, as far as I can tell there are simply fewer people who use 'reoccur' instead, which is a newer back-formation (from re- + occurrence) than recur, which comes from the Latin.
    – z7sg Ѫ
    Commented Jul 12, 2011 at 14:20
8

To get the feel of it, try going into the negative:

-- "We don't want this to reoccur!" means that we don't want it to ever happen again.

-- "We don't want this to recur!" means that we don't want it to happen repeatedly at regular intervals, but randomly and sporadically is OK.

Improve this answer
1
  • Very interesting.
    – WAF
    Commented Jul 12, 2011 at 17:17
6

Does this rule exist, based on usage?

That's an awfully loaded question, so for a loaded answer we can ask Google for an NGram:

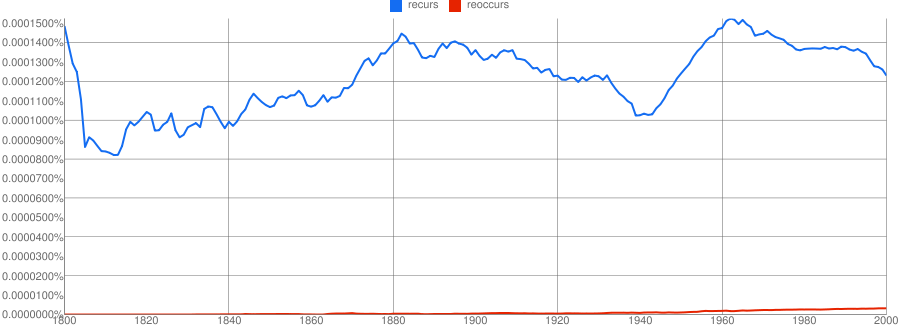
NGram of "recurs" vs "reoccurs"

As you can see, "reoccurs" is very uncommon compared with "recurs", when the rule would suggest we should see it more of the time. We get much the same picture graphing "reoccur" against "recur". This tends to suggest that we would be on very dodgy ground basing this rule on usage.

Does it mean the rule is wrong? Well, no. Consider:

Also, according to this distinction, is the first recurrence of something strictly a reoccurence and not a recurrence? That seems a little incredible.

What you are missing (and what doesn't show up in the NGrams) is that the first reoccurence is also a recurrence. Two data points do make a (trivial) repeated pattern, in exactly the same way that you can draw a straight line through any two points on a graph.

Notice also that something that recurs only perhaps happens at regular intervals. As you said, this is a matter of nuanced meaning, not hard and fast rule.

In summary, in theory we could use recurs and reoccurs to draw the distinction between regular and irregular repetition, but in practice we don't use reoccurs often enough for the distinction to be obvious to readers.

Improve this answer
5
  • Your explanation makes sense, and according to it the description of the distinction that I quoted is at best poorly worded and at worst misleading.
    – WAF
    Commented Jul 12, 2011 at 14:23
  • @WAF: on the contrary, I would say that the distinction is very carefully worded, just very subtle.
    – user1579
    Commented Jul 12, 2011 at 14:30
  • The qualifiers on the event's happening at regular intervals are "perhaps" (for recur) and "not necessarily" (for reoccur). These terms are equivalent unless they express degrees of likelihood. Also "repeatedly" in that formulation must mean "at least three times", which is strange.
    – WAF
    Commented Jul 12, 2011 at 14:52
  • "Something that reoccurs happens again, but not necessarily repeatedly..." In this case, either the event of happening is repeated, in which case it must occur three times to be consistent with the definition, or the event of reoccurring is repeated, in which case this is either a truism, or a recursive definition.
    – WAF
    Commented Jul 12, 2011 at 15:12
  • @Rhodri let us continue this discussion in chat
    – WAF
    Commented Jul 12, 2011 at 15:36
2

I came here looking for and expecting to find that the two uses were broadly synonymous, and not even having an idea that there may be a difference with (actual or anticipated) repetition!

However, as I have been reading I am reminded of 'recursion' in my own field (of programming) where a routine calls itself; thus (potentially) re-running the same processing over and over. This meets the definition proposed of:

Something that recurs happens repeatedly, perhaps at regular intervals

Furthermore, the Wikipedia article on Recursion emphasises this aspect of repetition.

I appreciate that looking at meanings of words based on a similar root may not be basis of the best argument, but at least in so doing we can see some similarities with the proposed definition.

Improve this answer
1
  • 1
    Can't believe I never noticed the relationship to 'recursion'. I was probably thrown off by the backronym 'recurse'. The quoted definition seems to fit conceptually extremely well, where the intervals are iterations rather than time per se.
    – WAF
    Commented Jan 22, 2015 at 13:01
-1

@jimreed is on the scent. Most English speakers are familiar with 'occur' etc., so when they need a word for the same thing happening more than once, they just follow standard (linguistic) English custom and put 're' in front of occur etc.

Latin isn't taught much in schools today, so 're' in front of 'cur' ;-) isn't really an option that could occur to most people.

Improve this answer

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .